SEAMIC_Integrals: Gamma Function

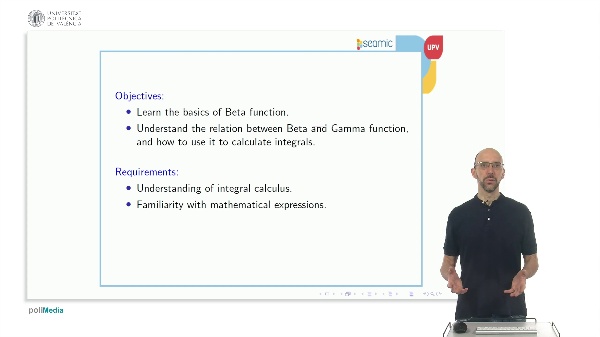
In this video we explore the Gamma function, its properties, and applications in calculus. We start by recalling that the exponential function approaches zero as x approaches infinity and that Gamma(1) = 1. The recurrence property of the Gamma function is introduced, which states that ¿(p) = (p-1) × ¿(p-1), allowing us to calculate values of the Gamma function for natural numbers. We also learn that ¿(p) can be calculated using integration by parts and that it's a generalization of the factorial function. Additionally, we see how to apply these properties to solve integrals and calculate values of the Gamma function for non-natural numbers, including fractions, using the property ¿(1/2) = ¿¿.